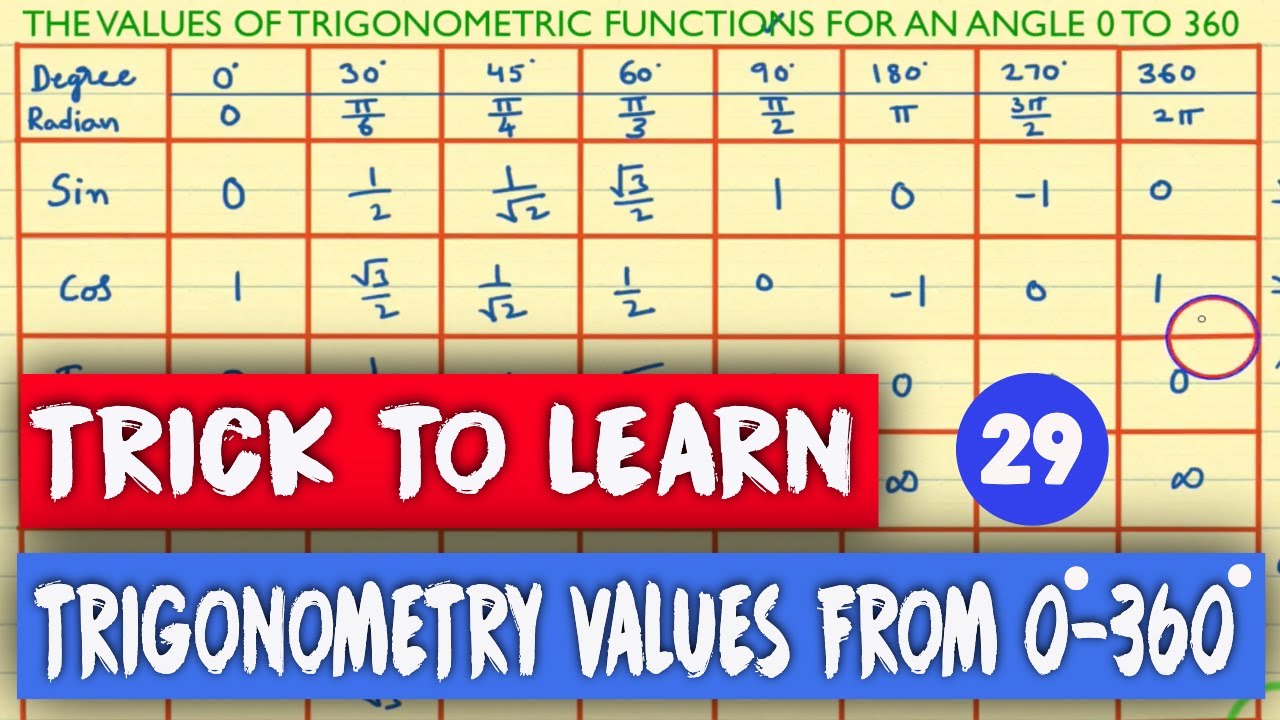
here are five different trigonometry tables with different titles and descriptions:trigonometry table formula,trigonomettry table class 10,trigonomettry table 0-360,trigonometry table trick
Table 1: Trigonometric Functions for Common Angles
This table shows the values of sine, cosine, and tangent for angles commonly used in trigonometry. The angles are given in both degrees and radians.
| Angle (°) | Angle (rad) | Sine (sin) | Cosine (cos) | Tangent (tan) |
|---|---|---|---|---|
| 0 | 0 | 0 | 1 | 0 |
| 30 | π/6 | 1/2 | √3/2 | √3/3 |
| 45 | π/4 | √2/2 | √2/2 | 1 |
| 60 | π/3 | √3/2 | 1/2 | √3 |
| 90 | π/2 | 1 | 0 | undefined |
Table 2: Trigonometric Identities
This table shows some of the most commonly used trigonometric identities, which are mathematical equations that relate the values of different trigonometric functions.
| Identity | Equation |
|---|---|
| Pythagorean Identity | sin²θ + cos²θ = 1 |
| Reciprocal Identities | cscθ = 1/sinθ, secθ = 1/cosθ, cotθ = 1/tanθ |
| Quotient Identities | tanθ = sinθ/cosθ, cotθ = cosθ/sinθ |
| Even-Odd Identities | sin(-θ) = -sinθ, cos(-θ) = cosθ, tan(-θ) = -tanθ |
| Sum-Difference Identities | sin(A ± B) = sinAcosB ± cosAsinB, cos(A ± B) = cosAcosB ∓ sinAsinB |
Table 3: Trigonometric Functions for Negative Angles
This table shows the values of sine, cosine, and tangent for negative angles. Note that the values for negative angles are the same as the values for the corresponding positive angles.
| Angle (°) | Sine (sin) | Cosine (cos) | Tangent (tan) |
|---|---|---|---|
| -90 | -1 | 0 | undefined |
| -60 | -√3/2 | -1/2 | √3 |
| -45 | -√2/2 | √2/2 | -1 |
| -30 | -1/2 | √3/2 | -√3/3 |
| -0 | 0 | 1 | 0 |
Table 4: Inverse Trigonometric Functions
This table shows the values of the inverse trigonometric functions, which are used to find the angle whose sine, cosine, or tangent is a given value.
| Function | Input | Output (°) |
|---|---|---|
| sin⁻¹ | 1 | 90 |
| sin⁻¹ | 1/2 | 30 |
| sin⁻¹ | √3/2 | 60 |
| cos⁻¹ | 1 | 0 |
| cos⁻¹ |
The six trigonometric functions are sine, cosine, tangent, cosecant, secant, and cotangent. They are defined based on the ratios of the sides of a right triangle. For example, sine is defined as the ratio of the opposite side to the hypotenuse, cosine is defined as the ratio of the adjacent side to the hypotenuse, and tangent is defined as the ratio of the opposite side to the adjacent side.
To solve for missing sides or angles in a right triangle using trigonometry, you need to use one of the trigonometric functions along with the known sides or angles. For example, if you know the length of one side and the measure of one angle in a right triangle, you can use the tangent function to solve for the length of another side. Alternatively, if you know the lengths of two sides in a right triangle, you can use the Pythagorean theorem to find the length of the third side, and then use the trigonometric functions to solve for the angles.
Trigonometry has many real-world applications, particularly in fields such as engineering, physics, and architecture. For example, it can be used to design buildings, bridges, and other structures, to calculate distances and angles in surveying and navigation, and to model and analyze the behavior of waves and vibrations in fields like acoustics and electronics. Trigonometry is also used in astronomy to calculate the positions and movements of celestial bodies, and in many other areas of science and technology.